Quadratic Functions - Mrs.Mathpedia

A quadratic function is a polynomial of degree 2 which has the form

$f (x) = a x^{2} + b x + c$

where $x$ is an unknown and where $a, b$ and $c$ are constants with $a \neq 0$ .

Contents [hide]

1 Quadratic Formula
2 The number of solutions
3 Graphs
4 Inequality

Quadratic Formula

The quadratic formula provides the solutions to a quadratic equation. $a x^{2} + b x + c = 0$ where $a \neq 0$ . That is, the quadratic equation has two solutions:

$x = \frac{- b \pm \sqrt{b^{2} - 4 a c}}{2 a}$

This formula indicates that can be factored into as below:

$a x^{2} + b x + c = a (x - \frac{- b + \sqrt{b^{2} - 4 a c}}{2 a}) (x - \frac{- b - \sqrt{b^{2} - 4 a c}}{2 a}) = 0$

The geometric interpretation is that $y = a x^{2} + b x + c$ intersects with $x$ -axis at $(\frac{- b - \sqrt{b^{2} - 4 a c}}{2 a}, 0)$ and $(\frac{- b + \sqrt{b^{2} - 4 a c}}{2 a}, 0)$ if $b^{2} - 4 a c \geq 0$ .

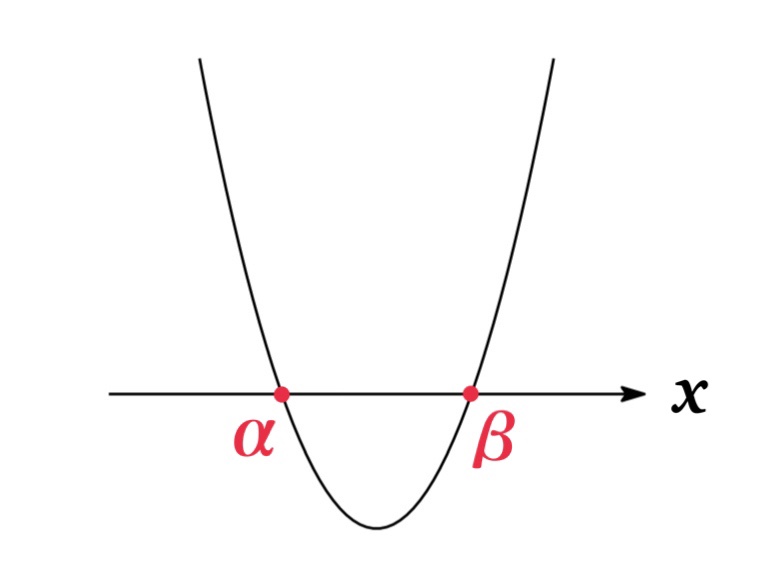
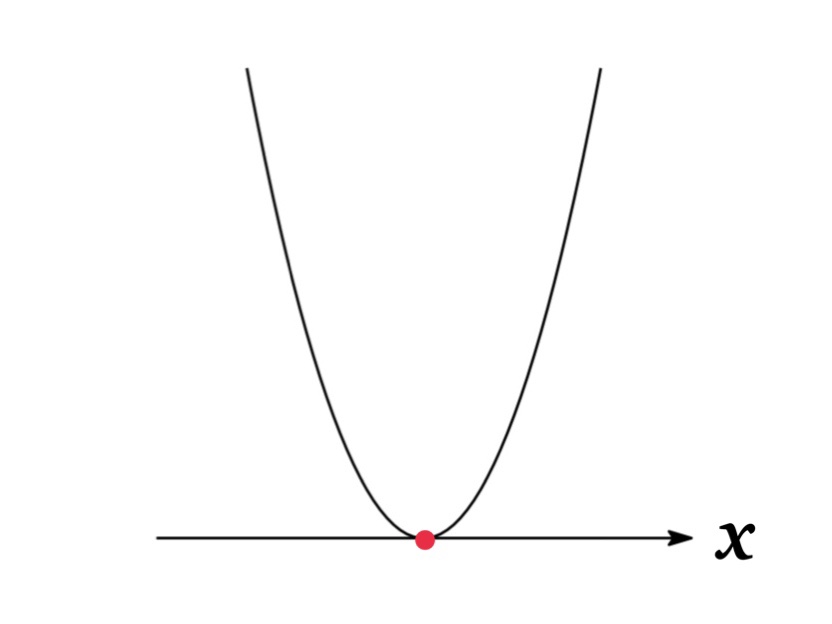
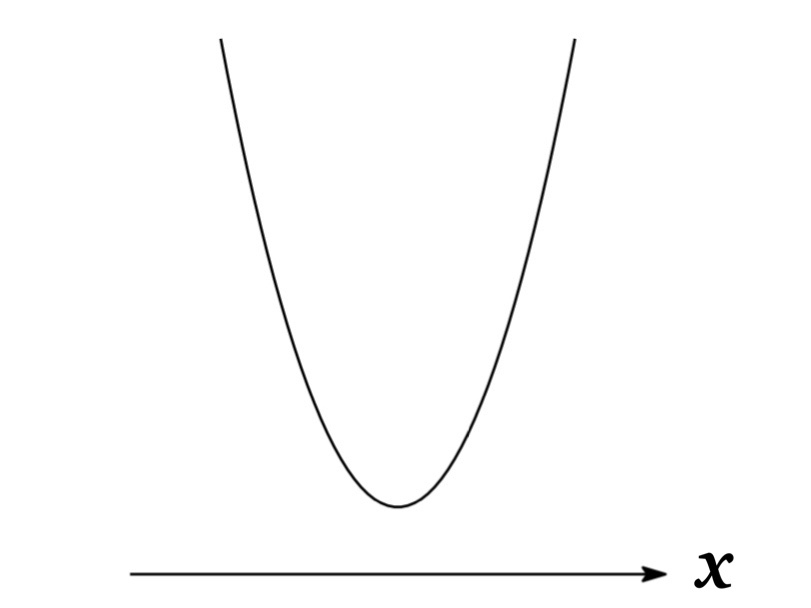
$α = (\frac{- b - \sqrt{b^{2} - 4 a c}}{2 a}, 0)$ , $β = (\frac{- b + \sqrt{b^{2} - 4 a c}}{2 a}, 0)$

The number of solutions

	Discriminant	Intersections of $y = a x^{2} + b x + c$ and $x$ axis	Solutions of $a x^{2} + b x + c = 0$
Example 1	$b^{2} - 4 a c > 0$	two intersections	Distinct two solutions: $x = \frac{- b - \sqrt{b^{2} - 4 a c}}{2 a}, \frac{- b + \sqrt{b^{2} - 4 a c}}{2 a}$
Example 2	$b^{2} - 4 a c = 0$	one intersection	Repeated root: $x = \frac{- b}{2 a}$
Example 3	$b^{2} - 4 a c < 0$	no intersection	None in real numbers: $x = \frac{- b - \sqrt{(4 a c - b^{2})} i}{2 a}, \frac{- b + \sqrt{(4 a c - b^{2})} i}{2 a}$

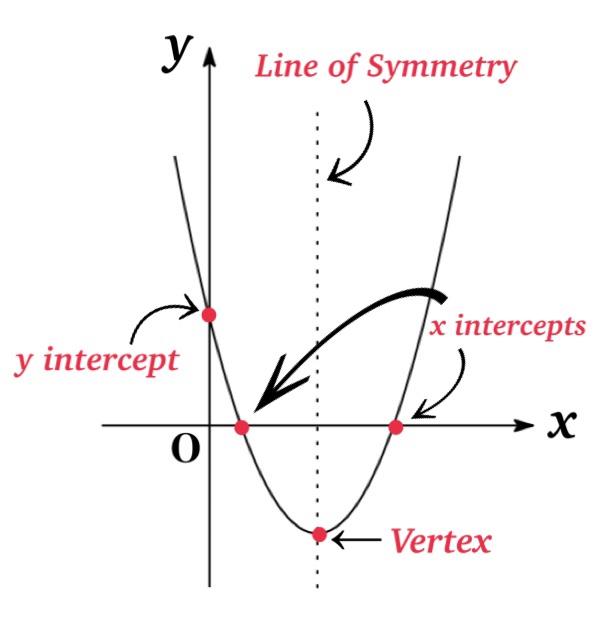
Graphs

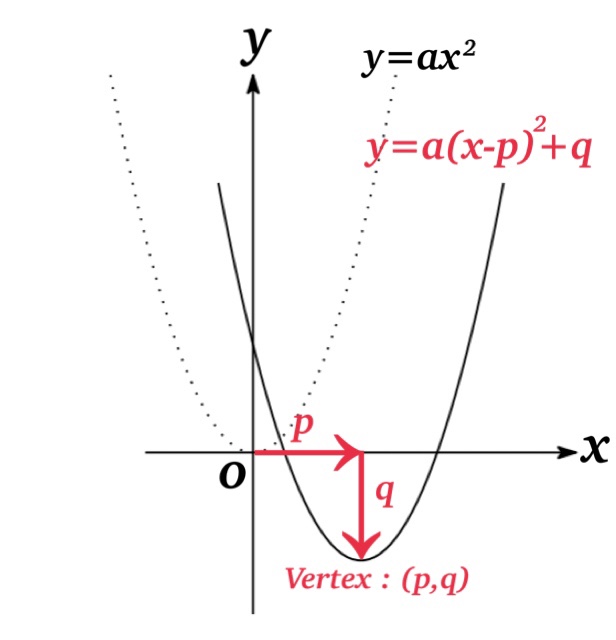
The shape of $y = a x^{2} + b x + c$ is called parabola and the leading coefficient, $a$ , determine the parabola opens upward or downward.

As shown in Fig, if $a$ is positive, the parabola opens upward, and if $a$ is negative, the parabola opens downward.

y = a x^{2} + b x + c

can be rewrite as

$a x^{2} + b x + c = a {(x + \frac{b}{2 a})}^{2} - \frac{b^{2} - 4 a c}{4 a}$

and this will tell us the vertex and the vertical line of symmetry.


$x$ -intercepts	$(\frac{- b - \sqrt{b^{2} - 4 a c}}{2 a}, 0)$ and $(\frac{- b + \sqrt{b^{2} - 4 a c}}{2 a}, 0)$
$y$ -intercept	$(0, c)$
Vertex	$(\frac{b}{2 a}, - \frac{b^{2} - 4 a c}{4 a})$
Line of Symmetry	$x = - \frac{b}{2 a}$

Notice that not all quadratic function have intercepts, but only quadratic functions whose discriminants is $D \geq 0$ have two intercepts or one intercept.

Furthermore, the graph of $y = a x^{2} + b x + c$ is given by shifting the graph $y = a x^{2}$ horizontally $- \frac{b}{2 a}$ and vertically $- \frac{b^{2} - 4 a c}{4 a}$ .

Inequality

Let $α$ and $β$ be the solutions to $a x^{2} + b x + c = 0$ . Then $α$ and $β$ are the intersections of $y = a x^{2} + b x + c$ and $x$ axis, the solutions to inequality are given as below:

$a > 0$	Solutions
$a x^{2} + b x + c > 0$	$x < α, β < x$
$a x^{2} + b x + c < 0$ .	$α < x < β$

$a < 0$	Solutions
$a x^{2} + b x + c > 0$	$α < x < β$
$a x^{2} + b x + c < 0$ .	$x < α, β < x$

Others

href="https://mrs-mathpedia.com/">Mrs.Mathpedia

Quadratic Formula

The number of solutions

Graphs

Inequality